The dynamics of motion, particularly linear and angular acceleration, form the cornerstone of understanding movement in the physical world. These two types of acceleration, although distinct in their nature, are fundamentally linked in ways that allow for the intricate movements observed in everything from the smallest particles to the largest celestial bodies. This relationship underpins a variety of phenomena in physics and engineering, providing insights into how objects move and interact within their environments.
Linear acceleration refers to the change in velocity of an object moving along a straight path, while angular acceleration describes the change in angular velocity of an object’s rotation. The key connection between these concepts lies in their mutual dependence on force and torque, respectively, and how these influences can be mathematically related through the radius of rotation. This relationship is pivotal in converting between linear and angular measures, enabling precise predictions and analyses of motion in diverse applications.
Understanding the link between linear and angular acceleration is not just an academic exercise; it has practical implications across a wide array of fields, from automotive engineering to robotics, and from sports science to aerospace technology. By examining how these accelerations interact and influence each other, one gains valuable insights into designing more efficient machinery, improving athletic performance, and even in the development of technologies for space exploration.
Basics of Motion
Motion is a fundamental concept in physics that describes the change in position of an object over time. It’s divided into two main types: linear and angular motion, each with its unique properties, applications, and governing principles.
Linear Acceleration
Definition and formula
Linear acceleration refers to the rate of change of velocity of an object in motion along a straight path. It is quantified as the change in velocity per unit of time and is expressed in meters per second squared (m/s²). The formula for linear acceleration (a) is given by a = Δv / Δt, where Δv is the change in velocity and Δt is the change in time.
Examples in daily life
Everyday instances of linear acceleration are ubiquitous and simple to relate to. For example:
- When a car speeds up from a stoplight, it is experiencing linear acceleration.
- An elevator starting its ascent or slowing down before stopping demonstrates linear acceleration.
- Throwing a ball, where its speed increases as it leaves your hand, is another illustration.
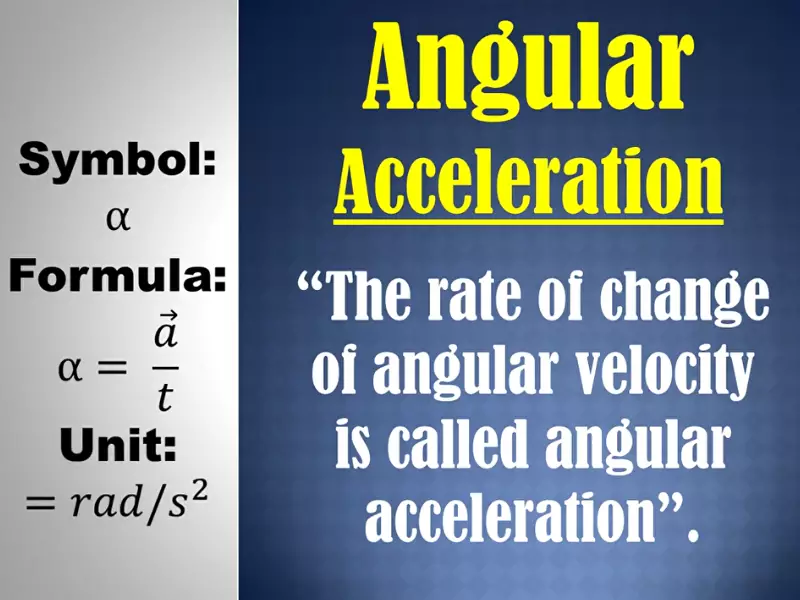
Angular Acceleration
Definition and formula
Angular acceleration is the rate of change of angular velocity of an object rotating about an axis. It is measured in radians per second squared (rad/s²). The formula for angular acceleration (α) is α = Δω / Δt, where Δω is the change in angular velocity and Δt is the change in time.
Examples in real-world scenarios
Angular acceleration is not as visibly apparent as linear acceleration but plays a crucial role in various real-world applications, such as:
- The spinning of a Ferris wheel, where its speed of rotation increases or decreases.
- Disc brakes in a vehicle apply angular acceleration to slow down or stop the wheel’s rotation.
- A figure skater spinning faster by pulling their arms inwards is exploiting angular acceleration.
Core Principles
The understanding of motion, both linear and angular, is deeply rooted in Newton’s laws of motion and the principles of rotational dynamics.
Newton’s Laws of Motion
Brief overview
Isaac Newton’s three laws of motion form the foundation for classical mechanics, explaining the relationship between a body and the forces acting upon it, and its motion in response to those forces.
Relevance to acceleration
These laws are crucial for understanding how and why objects accelerate. For instance:
- Newton’s first law (Law of Inertia) explains why a body maintains its state of rest or uniform motion unless acted upon by an external force, influencing both linear and angular acceleration.
- Newton’s second law connects force, mass, and acceleration, stating that
F = mafor linear motion, and analogously,τ = Iαfor rotational motion, whereτis torque andIis the moment of inertia. - Newton’s third law (Action and Reaction) is observable in scenarios where forces result in equal and opposite reactions, affecting acceleration.
Rotational Dynamics
Moment of inertia
The moment of inertia (I) is a measure of an object’s resistance to changes in its rotation. It depends on the object’s mass distribution relative to the axis of rotation. Higher moments of inertia mean more resistance to angular acceleration.
Torque and its effect
Torque (τ) is the rotational equivalent of force. It is the product of force and the perpendicular distance from the axis of rotation to the point where the force is applied. Torque plays a pivotal role in determining an object’s angular acceleration.
Linking Linear and Angular Acceleration
Understanding the connection between linear and angular acceleration is vital for grasping the comprehensive nature of motion.
The Relationship Explained
Linear and angular acceleration are intertwined through the radius of the path of motion. An object moving in a circular path experiences both types of acceleration, with linear acceleration directed tangentially and angular acceleration directed radially.
Mathematical connection
The mathematical link between the two can be represented by the equation a = rα, where a is the linear acceleration, α is the angular acceleration, and r is the radius of the circular path.
Radius of rotation’s role
The radius of rotation significantly impacts the relationship between linear and angular acceleration. A larger radius means that for the same angular acceleration, the linear acceleration will be greater, illustrating the proportional relationship between the two.
Key Formulas
The mathematical framework for understanding motion includes crucial formulas that bridge linear and angular dynamics.
Derivation of formulas
By exploring the derivations of key formulas, we gain insights into the fundamental principles that govern motion. For instance, the formula a = rα derives from equating the linear velocity to the product of angular velocity and radius, then differentiating with respect to time.
Interconversion between linear and angular acceleration
This principle allows for the conversion of linear acceleration measurements into angular acceleration figures and vice versa, facilitating a comprehensive analysis of motion in various contexts.
Practical Applications
The principles of motion find extensive application in engineering, design, sports science, and more, illustrating the universal nature of these concepts.
Engineering and Design
Machinery and gear systems
In machinery and gear systems, understanding the relationship between linear and angular acceleration ensures efficient operation, minimal wear, and optimized design for performance and safety.
Automotive applications
In the automotive industry, from the mechanism of acceleration in vehicles to the design of brakes, the principles of linear and angular acceleration are applied to enhance functionality, safety, and performance.
Sports Science
Analysis of human motion
The study of human motion, particularly in sports, benefits from an understanding of linear and angular acceleration. It aids in improving athletic performance, designing better training regimens, and preventing injuries.
Equipment design
Sports equipment design, from golf clubs to racing cars, relies on the principles of motion to optimize performance, safety, and user experience, demonstrating the far-reaching applications of these fundamental concepts.

Advanced Topics
The study of motion extends into more complex areas that deepen our understanding of physical phenomena and their applications.
Non-Uniform Circular Motion
Variations in acceleration
In non-uniform circular motion, the speed of an object traveling along a circular path changes, leading to both tangential and radial (centripetal) acceleration. This dual aspect of acceleration results from changes in the magnitude of velocity (linear acceleration) and changes in direction (centripetal acceleration).
Impact on object dynamics
The dynamics of an object undergoing non-uniform circular motion are significantly affected by these variations in acceleration. It leads to complex motion patterns and requires careful analysis to predict movement accurately. This is critical in designing roller coasters, where ensuring passenger safety depends on understanding these dynamics.
Angular Momentum
Conservation principles
Angular momentum, the rotational equivalent of linear momentum, is conserved in a closed system where no external torques are applied. This principle of conservation plays a crucial role in phenomena ranging from the spin of a figure skater to the orbit of celestial bodies.
Relation with angular acceleration
Angular momentum is directly related to angular velocity and, consequently, angular acceleration. An object’s moment of inertia and angular velocity determine its angular momentum. Therefore, any change in angular acceleration affects angular momentum, provided the system’s moment of inertia remains constant.
Common Misunderstandings
Even within the scientific community, misconceptions can arise, especially when differentiating between closely related concepts.
Clarification of misconceptions
One common misunderstanding is the confusion between angular speed and angular acceleration. While angular speed measures how fast an object rotates, angular acceleration measures how quickly the rotation speed changes.
Difference between speed and acceleration in circular motion
Another frequent confusion lies in equating speed with acceleration in circular motion. Speed refers to the distance an object travels per unit of time, whereas acceleration refers to the rate of change of speed or direction. In circular motion, even if the speed is constant, the object accelerates because its direction changes continuously.
Calculation Examples
To elucidate these concepts, let’s explore how to calculate linear and angular acceleration through practical examples.
Step-by-step guide on calculating linear and angular acceleration
Calculating Linear Acceleration:
- Identify the initial and final velocities (
v_iandv_f) of the object. - Determine the time interval (
Δt) during which the change occurs. - Use the formula
a = (v_f - v_i) / Δtto find the linear acceleration.
Calculating Angular Acceleration:
- Determine the initial and final angular velocities (
ω_iandω_f). - Identify the time interval (
Δt) over which the change happens. - Apply the formula
α = (ω_f - ω_i) / Δtto calculate the angular acceleration.
Practical problems and solutions
Example: A car accelerates from rest to 60 km/h in 5 seconds. What is its linear acceleration?
- Convert 60 km/h to m/s: 60 km/h = 16.67 m/s.
- Initial velocity (
v_i) = 0 m/s, final velocity (v_f) = 16.67 m/s, time (Δt) = 5 s. - Linear acceleration (
a) = (16.67 – 0) / 5 = 3.33 m/s².
Example: A disk starts from rest and reaches an angular velocity of 10 rad/s in 2 seconds. Calculate its angular acceleration.
- Initial angular velocity (
ω_i) = 0 rad/s, final angular velocity (ω_f) = 10 rad/s, time (Δt) = 2 s. - Angular acceleration (
α) = (10 – 0) / 2 = 5 rad/s².
Impact on Technology and Innovation
The principles of linear and angular acceleration have profound impacts on modern technology and innovation.
Advances in Robotics
Precision in movement and control
In robotics, precise control over linear and angular acceleration is crucial for achieving smooth and accurate movements. This precision enables robots to perform complex tasks in manufacturing, surgery, and exploration.
Aerospace Developments
Satellites and space exploration mechanics
The principles of motion, particularly angular momentum and acceleration, are fundamental in designing and controlling satellites and spacecraft. Understanding these principles allows for the accurate positioning of satellites and the planning of space missions, contributing to advancements in communication, exploration, and scientific research.
Frequently Asked Questions
How do linear and angular acceleration relate?
Linear and angular acceleration are related through the radius of the object’s path of motion. Mathematically, linear acceleration is equal to the angular acceleration multiplied by the radius of rotation. This relationship allows for the conversion between linear and angular measures, crucial for understanding and designing systems involving rotational motion.
Can you have angular acceleration without linear acceleration?
Yes, it is possible to have angular acceleration without linear acceleration in scenarios where an object rotates about a fixed axis without changing its position in space. Examples include a spinning wheel or a planet rotating on its axis, where the angular velocity changes but the object does not move linearly through space.
What role does torque play in angular acceleration?
Torque is the rotational equivalent of force and plays a pivotal role in angular acceleration. It is the torque applied to an object that causes it to have angular acceleration. The amount of torque required for a certain angular acceleration depends on the object’s moment of inertia, which is a measure of how much resistance it offers to changes in its rotational motion.
Conclusion
The intricate dance between linear and angular acceleration is a fundamental aspect of motion that drives the physical world around us. Through understanding this relationship, we unlock the potential to innovate, design, and improve upon the mechanical and technological solutions that shape our daily lives. Whether it’s enhancing the performance of vehicles, advancing robotics, or pushing the boundaries of space exploration, the principles governing linear and angular acceleration remain at the heart of progress.
This exploration not only broadens our comprehension of motion but also underscores the interconnectedness of physical laws in shaping the universe. As we continue to delve into the mysteries of motion, the insights gained from understanding the relation between linear and angular acceleration will undoubtedly continue to propel forward the frontiers of science and engineering, paving the way for future discoveries and innovations.
