Kinetic energy and momentum are foundational concepts in physics, each playing a critical role in understanding the motion of objects. While kinetic energy relates to the energy an object possesses due to its motion, momentum measures the ‘quantity’ of that motion, taking into account both the object’s mass and velocity. These concepts not only help in predicting the outcomes of physical interactions but also in solving complex problems across various scientific and engineering domains.
The relationship between kinetic energy and momentum is not just a matter of academic interest but a cornerstone of classical mechanics. Essentially, while kinetic energy gives us an understanding of the work an object can perform due to its motion, momentum helps us understand how much force is needed to change that motion. This duality is crucial for accurately describing the physical world around us.
Exploring the nuances of these relationships reveals deeper insights into energy conservation, collision dynamics, and even quantum mechanics. By examining how kinetic energy and momentum interplay, one can predict the aftermath of collisions, design safer vehicles, and even navigate the depths of space more effectively. This interrelation is pivotal in both theoretical physics and practical applications, guiding advancements in technology and our understanding of the universe.

Kinetic Energy Explained
Definition
Kinetic energy is the energy an object possesses due to its motion. It’s a fundamental concept in physics, highlighting how objects in motion carry energy capable of doing work—from powering machines to causing changes in other objects upon impact. The basic premise is: the faster something moves, the more kinetic energy it has.
Calculation Formula
The formula for calculating kinetic energy (KE) is:
��=12��2KE=21mv2
where:
- �m stands for mass of the object (in kilograms),
- �v represents the velocity of the object (in meters per second),
- ��KE is the kinetic energy (in joules).
This equation reveals two critical factors: mass and velocity. It shows that kinetic energy increases with the square of the velocity, indicating that speed has a more profound effect on kinetic energy than mass.
Factors Affecting Kinetic Energy
Several factors influence an object’s kinetic energy, primarily its mass and velocity:
- Mass: A heavier object will have more kinetic energy if it moves at the same speed as a lighter one.
- Velocity: An increase in speed significantly boosts kinetic energy due to the velocity’s squared relationship in the formula.
Understanding these factors is crucial for applications ranging from engineering projects to everyday tasks, emphasizing the importance of both mass and velocity in kinetic energy’s practical effects.
Momentum Unveiled
Definition
Momentum is a measure of an object’s tendency to keep moving at a constant velocity. It’s a vector quantity, meaning it has both magnitude and direction. Momentum helps us understand how difficult it is to stop a moving object or change its course. It’s a key concept in physics, encapsulating the quantity of motion an object has.
Calculation Formula
The formula for momentum (�p) is:
�=��p=mv
where:
- �m is the mass of the object (in kilograms),
- �v is its velocity (in meters per second),
- �p represents momentum (in kilogram meters per second).
This equation highlights the direct relationship between an object’s mass, its velocity, and its momentum. Unlike kinetic energy, momentum’s dependence on velocity is linear, making both mass and velocity equally significant in determining an object’s momentum.
Factors Influencing Momentum
Momentum is affected by two main factors: mass and velocity:
- Mass: A greater mass means more momentum if the velocity is constant. This is why heavy vehicles, like trucks, have more momentum at the same speed as a car.
- Velocity: An object moving faster will have more momentum. This explains why even a small bullet can have significant momentum when fired from a gun.
Understanding these factors is essential in fields like vehicle safety design, where managing momentum can mean the difference between a minor incident and a catastrophic collision.
Kinetic Energy vs Momentum
Basic Differences
While both kinetic energy and momentum relate to an object’s motion, they differ fundamentally:
- Kinetic Energy is a scalar quantity related to an object’s speed and mass, focusing on the energy due to motion.
- Momentum is a vector quantity, involving both the mass and velocity of an object, emphasizing the quantity of motion.
The Relationship
Kinetic energy and momentum are interconnected yet serve different conceptual purposes. Kinetic energy measures the work an object can do because of its motion, while momentum quantifies its motion’s persistence or resistance to stopping.
Mathematical Connection
There’s a mathematical connection between kinetic energy and momentum, particularly evident in their formulas. While kinetic energy is proportional to the square of velocity, momentum is linearly related. This distinction highlights different aspects of motion’s impact.
Deriving the Relationship
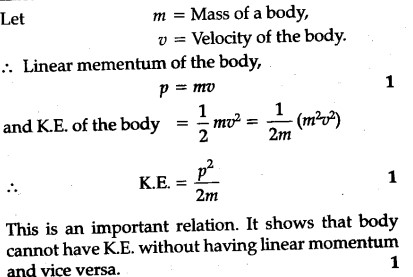
The relationship between kinetic energy and momentum can be further explored by manipulating their formulas. For instance, kinetic energy can be expressed in terms of momentum as:
��=�22�KE=2mp2
where �p is momentum. This equation underscores how kinetic energy and momentum are intricately linked through mass and velocity, providing a deeper understanding of motion’s effects.
Example Calculations
To illustrate these concepts, consider example calculations for both kinetic energy and momentum:
- Kinetic Energy Example: A car with a mass of 1000 kg moving at 20 meters per second has a kinetic energy of:
��=12×1000×202=200,000 joulesKE=21×1000×202=200,000 joules
- Momentum Example: The same car will have a momentum of:
�=1000×20=20,000 kg m/sp=1000×20=20,000 kg m/s
These examples highlight how kinetic energy and momentum provide different perspectives on motion’s implications, with kinetic energy focusing on the energy aspect and momentum on the motion’s persistence.
Impact on Motion
In Collisions
Kinetic Energy and Momentum
Collisions involve two key principles: kinetic energy and momentum. The role they play is crucial in understanding the outcomes of such events. Momentum is always conserved in collisions, regardless of whether they are elastic or inelastic. However, kinetic energy behaves differently based on the type of collision.
- Elastic Collisions: Both momentum and kinetic energy are conserved. This is seen in ideal scenarios, such as in billiard balls striking each other, where the motion after impact is a direct result of both conserved quantities.
- Inelastic Collisions: Momentum is conserved, but kinetic energy is not fully conserved. Some of it is transformed into other forms of energy, like heat or sound. Car crashes are common examples, where the deformation of the vehicles is a sign of kinetic energy transformation.
Understanding the dynamics of collisions is essential in fields like automotive safety, where designing vehicles to effectively manage energy and momentum can significantly improve crashworthiness.
In Energy Transfer
The Role of Kinetic Energy
Kinetic energy’s transformation during motion plays a pivotal role in energy transfer processes. It’s the mechanism behind various natural phenomena and technological applications, from the simple act of throwing a ball to the complex operations of a hydroelectric power plant. The ability of moving objects to do work—by transferring kinetic energy to other objects or forms of energy—is a fundamental concept in physics and engineering.
Real-World Applications
Engineering
In engineering, understanding kinetic energy and momentum is critical for designing machines and structures that can withstand dynamic forces. For instance, in building bridges, engineers must account for the kinetic energy and momentum of moving vehicles to ensure structural integrity and safety.
Sports Science
Sports science leverages these concepts to enhance athletic performance and prevent injuries. Analyzing the kinetic energy and momentum in movements can help athletes optimize their motions for maximum efficiency and power, while also understanding how to reduce the impact forces on their bodies.
Space Exploration
Space exploration missions depend heavily on kinetic energy and momentum. Spacecraft use the conservation of momentum for navigation, employing maneuvers like gravity assists to gain speed without expending fuel. Additionally, understanding kinetic energy is crucial for managing the impacts of micrometeoroids on spacecraft surfaces.
Enhancing Understanding
Experiment Ideas
Hands-on experiments are a fantastic way to grasp kinetic energy and momentum’s principles:
- Pendulum Experiments: Observing pendulums can help visualize how kinetic energy converts into potential energy and back, illustrating energy conservation.
- Collision Experiments: Using balls of different masses and materials, one can investigate how momentum is conserved in various types of collisions, highlighting the difference between elastic and inelastic collisions.
Simulation Tools
Simulation tools offer a modern approach to studying kinetic energy and momentum. Software like PhET Interactive Simulations allows users to model and manipulate variables in virtual environments, providing a deeper understanding of the concepts without the need for physical materials or extensive setup.
Common Questions
FAQs on Kinetic Energy and Momentum
- How is kinetic energy different from potential energy? Kinetic energy is related to an object’s motion, while potential energy is related to its position or state. Potential energy can convert into kinetic energy and vice versa, but they highlight different energy forms.
- Can an object have momentum without kinetic energy? No, because both momentum and kinetic energy depend on the object’s mass and velocity. If an object is moving, it has both momentum and kinetic energy.
- Why is kinetic energy squared, but momentum is not? The squaring in the kinetic energy formula reflects how energy scales with the square of velocity, showing a more significant impact on energy from speed changes. Momentum’s linear relationship with velocity offers a different insight, emphasizing the combined effect of mass and velocity on motion persistence.
- What happens to the kinetic energy in an inelastic collision? In an inelastic collision, some kinetic energy is transformed into other energy forms, such as thermal energy or sound. This transformation is why objects in an inelastic collision don’t bounce back with the same energy they had before the impact.
Frequently Asked Questions
How are kinetic energy and momentum different?
Kinetic energy and momentum, though related, differ fundamentally in their dependence on velocity. Kinetic energy, expressed as 12��221mv2, increases with the square of an object’s velocity, indicating how energy scales with speed. In contrast, momentum, calculated as ��mv, varies directly with velocity, reflecting the mass and speed of an object in motion. This distinction highlights their different roles in understanding and predicting physical phenomena.
Can momentum exist without kinetic energy?
Momentum and kinetic energy are both products of an object’s mass and velocity, meaning neither can exist without the other in a moving object. However, their dependency on velocity differs—momentum linearly and kinetic energy quadratically. Therefore, any moving object with mass will inherently have both momentum and kinetic energy, with their magnitudes dependent on the object’s velocity and mass.
How does the conservation of momentum relate to kinetic energy?
The conservation of momentum principle states that in a closed system, the total momentum before and after an event remains constant. This principle often goes hand-in-hand with kinetic energy in collisions. In elastic collisions, both momentum and kinetic energy are conserved, allowing for the prediction of post-collision velocities. In inelastic collisions, while momentum is conserved, kinetic energy is not necessarily preserved, often converting into other forms of energy like heat or sound.
Conclusion
The intricate relationship between kinetic energy and momentum forms the bedrock of classical mechanics, providing a comprehensive framework for understanding motion and energy dynamics. These concepts not only illuminate the principles governing physical interactions but also offer practical insights into designing better machines, improving safety measures, and advancing our technological capabilities. Their application stretches far beyond theoretical physics, influencing real-world engineering, sports science, and space exploration.
In summary, kinetic energy and momentum are essential to grasping the complexities of motion in our universe. By exploring their relationship, one gains valuable perspectives on energy conservation, force application, and the fundamental laws that govern our physical world. Their study not only enriches our understanding of physics but also empowers innovation and progress across a multitude of scientific and technological fields.