The Nernst and Goldman equations are fundamental pillars in the study of biophysics and physiology, offering deep insights into the electrical properties of cells and their membranes. These equations enable scientists and researchers to calculate ion concentrations and membrane potentials, crucial for understanding cellular functions and neural communication. The distinction between these two equations lies in their scope and application, each serving as a tool for specific scenarios in electrochemistry and cellular physiology.
The Nernst equation provides a method to calculate the electrochemical equilibrium potential for a single ion type across a membrane, based on concentration gradients. In contrast, the Goldman equation extends this calculation to multiple ion types, accounting for their relative permeabilities and offering a more comprehensive view of membrane potentials. This comparative approach reveals the unique applications and significance of each equation in understanding cellular environments.
Both equations share a common goal: to elucidate the electrochemical dynamics of cells. The Nernst equation focuses on the equilibrium potential of individual ions, a fundamental concept for grasping how ions move across cell membranes. Meanwhile, the Goldman equation, by incorporating multiple ions, addresses the complexity of real cellular conditions, providing a closer approximation to actual membrane potentials. This distinction underscores their complementary roles in advancing our knowledge of cellular mechanisms.
Nernst Equation Basics
Overview
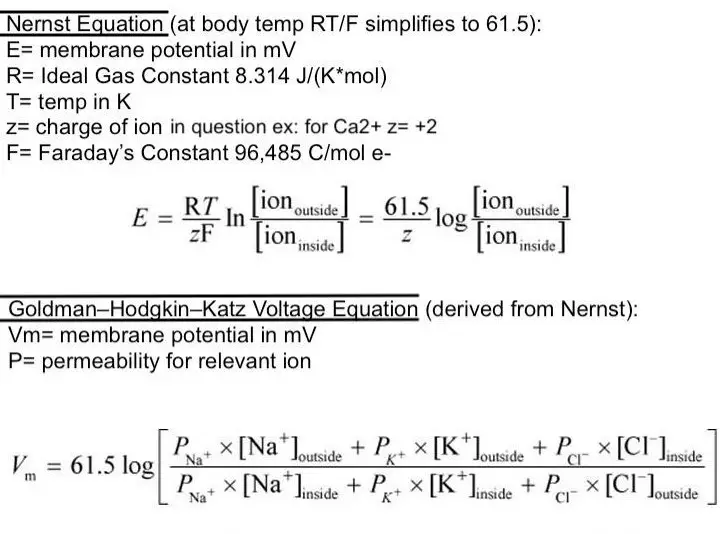
The Nernst equation stands as a cornerstone in electrochemistry, bridging chemistry and electricity to explain ion movement across membranes. Named after Walther Nernst, a Nobel Laureate, this equation has been a fundamental tool since its introduction in the late 19th century. Its role extends beyond mere calculation; it provides a window into the electrochemical equilibrium, crucial for understanding processes in batteries, neurons, and more.
Formula and Components
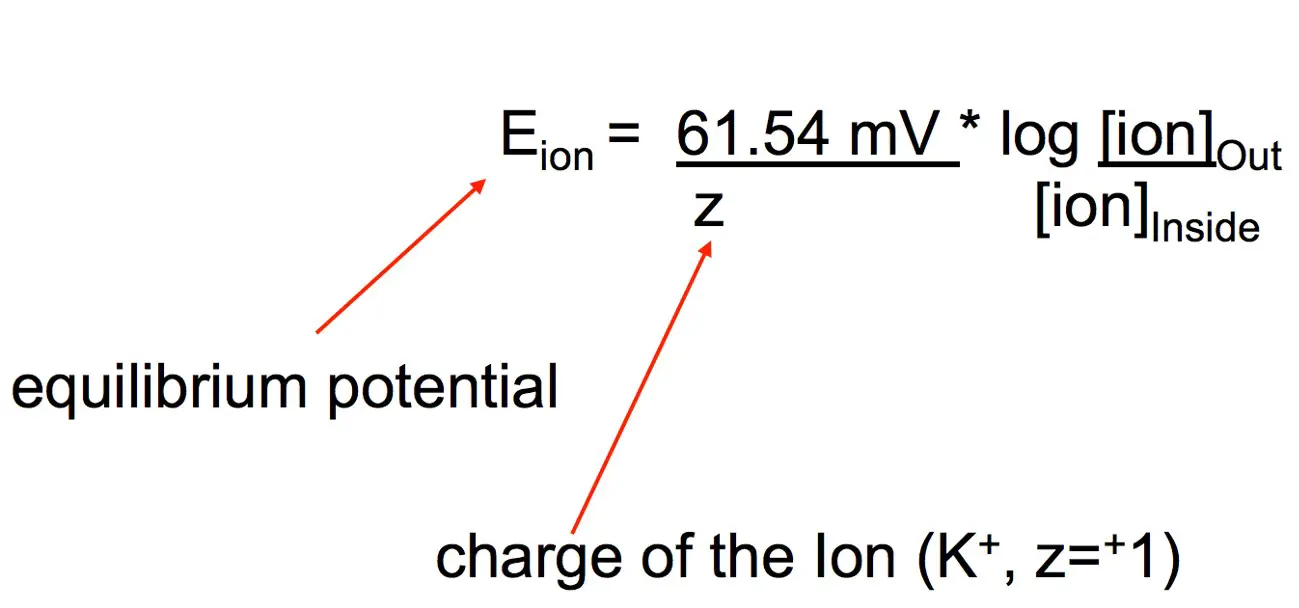
The Nernst equation is elegantly simple yet profound in its implications:
�=�0−����ln[����][���]E=E0−nFRTln[Cin][Cout]
- E is the membrane potential (voltage across the membrane).
- E^0 represents the standard electrode potential.
- R is the universal gas constant (8.314 J/(mol·K)).
- T stands for temperature in Kelvin.
- n is the number of moles of electrons transferred.
- F is the Faraday constant (approximately 96485 C/mol).
- [C_{out}] and [C_{in}] are the concentrations of the ion outside and inside the cell, respectively.
This formula encapsulates how ion concentration gradients drive membrane potentials, highlighting the interplay of chemistry and electricity at the cellular level.
Applications
The Nernst equation finds applications in various fields, including:
- Neuroscience: Calculating the resting potentials of neurons.
- Medicine: Understanding drug actions on ion channels.
- Environmental science: Analyzing metal ion concentrations in soils and waters.
These applications underscore the equation’s versatility in deciphering electrochemical behaviors across disciplines.
Goldman Equation Explained
Overview
The Goldman equation builds on the Nernst foundation, introduced to address the complexity of real biological membranes that are permeable to multiple ions. Named after David E. Goldman, this equation marks a significant leap in our understanding of membrane physiology, offering insights into how cells maintain their internal environments amidst a sea of different ions.
Formula Breakdown
The Goldman equation takes a comprehensive approach, considering multiple ions:
��=���ln��+[�+]���+���+[��+]���+���−[��−]����+[�+]��+���+[��+]��+���−[��−]���Vm=FRTlnPK+[K+]in+PNa+[Na+]in+PCl−[Cl−]outPK+[K+]out+PNa+[Na+]out+PCl−[Cl−]in
- V_m is the membrane potential.
- P_{ion} represents the permeability for each ion (K+, Na+, Cl-).
- [Ion]_{out/in} indicates the ion concentration outside/inside the cell.
This equation reflects the dynamic nature of cellular environments, factoring in the relative permeabilities and concentrations of key ions.
Key Differences from Nernst
The Goldman equation differs from the Nernst equation in several ways:
- Multi-ion consideration: Accounts for multiple ions, providing a holistic view of membrane potentials.
- Relative permeability: Incorporates the permeability of each ion, adding another layer of complexity to the calculation.
These differences highlight the Goldman equation’s broader applicability in modeling real cellular conditions.
Comparative Analysis
Contextual Applications
Each equation serves best in specific contexts:
- Nernst equation: Ideal for single-ion analyses, such as examining individual ion effects on membrane potential.
- Goldman equation: More suited for complex systems where multiple ions influence the membrane potential simultaneously.
Impact on Scientific Research
The introduction and use of these equations have significantly advanced our understanding in fields like cellular physiology and neurobiology. They’ve provided frameworks for exploring how cells communicate and maintain homeostasis, influencing both theoretical research and practical applications.
Practical Examples
In real-world scenarios, these equations illuminate diverse phenomena:
- Nernst equation: Helps explain why neurons have a negative resting potential, critical for understanding neural excitability.
- Goldman equation: Offers insights into the action potentials that underpin nerve impulses, essential for brain and nerve function.
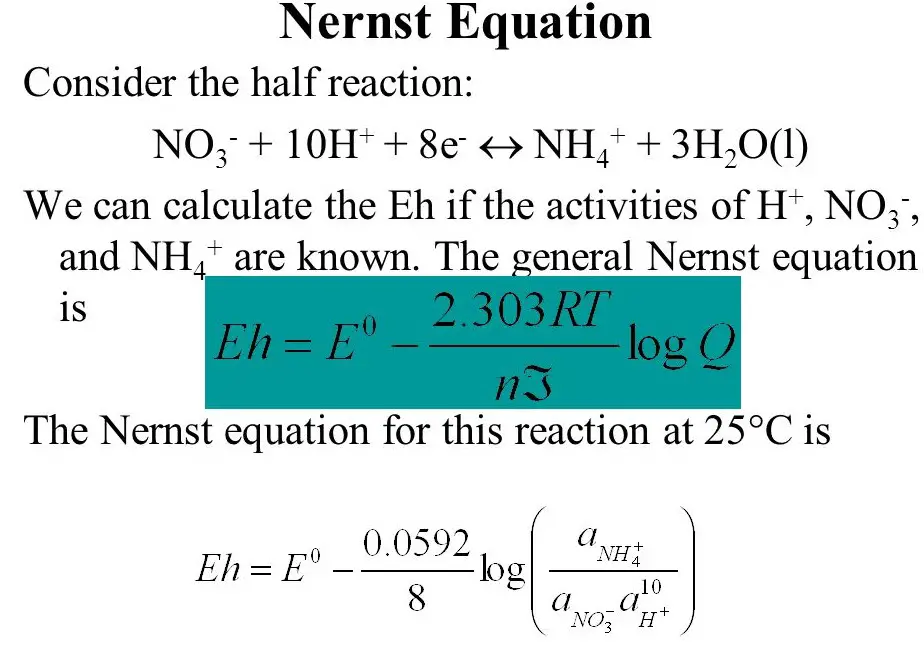
Advantages and Limitations
Nernst Equation Strengths
The Nernst equation is celebrated for its simplicity and precision in specific applications. Its strengths lie in its ability to:
- Provide exact values for the equilibrium potential of individual ions, crucial for understanding ion-specific movements across membranes.
- Serve as a foundational tool in electrochemistry, offering insights into battery design, electroplating, and corrosion prevention.
- Facilitate education in biochemistry and physics, where its straightforwardness helps demystify the electrical properties of cells.
This equation excels in scenarios where a clear, isolated view of a single ion’s behavior is needed, offering a lens through which the fundamental processes of electrochemical systems can be observed.
Nernst Equation Shortcomings
Despite its utility, the Nernst equation’s application comes with limitations:
- It cannot account for the combined effects of multiple ions which are common in biological systems, thus providing a simplified view of membrane potentials.
- Its reliance on the assumption of equilibrium may not always hold true in dynamic or non-equilibrium conditions frequently observed in living organisms.
These shortcomings underline the equation’s constraints in capturing the complex reality of cellular environments.
Goldman Equation Strengths
The Goldman equation stands out for its comprehensive approach to membrane potential calculation, characterized by:
- Its ability to integrate multiple ions and their permeabilities, offering a more holistic view of membrane potentials.
- Providing a closer approximation to the actual membrane potential in living cells, thereby enhancing the predictive power for physiological phenomena.
- Facilitating advanced neurological models, including those used in research on brain activity and neurodegenerative diseases.
Its strengths lie in its nuanced consideration of cellular complexity, making it indispensable in advanced physiological and biomedical research.
Goldman Equation Limitations
However, the Goldman equation is not without its challenges:
- Its complexity and requirement for detailed information about ion permeabilities can limit its accessibility and practicality in some educational and research settings.
- Potential inaccuracies in estimating ion permeabilities and concentrations can lead to errors in calculated membrane potentials, especially in highly dynamic systems.
These limitations highlight the equation’s dependency on precise data and the difficulties in applying it to rapidly changing biological conditions.
Recent Advances
Technological Enhancements
Modern technology and research have significantly refined the use and understanding of the Nernst and Goldman equations:
- Computational models and simulation software have advanced, allowing for more accurate and detailed predictions of membrane potentials and ion movements.
- High-resolution imaging and spectroscopy techniques provide precise measurements of ion concentrations and permeabilities, enhancing the accuracy of these equations.
- Artificial intelligence and machine learning algorithms are beginning to be applied in predicting ion channel behavior, potentially revolutionizing how we understand and apply both equations.
These technological advancements support a deeper and more nuanced application of these fundamental equations in scientific research.
Theoretical Developments
Recent theoretical advances have expanded or challenged the traditional use of these equations:
- Quantum mechanical models of ion transport are beginning to offer insights that might lead to revisions or extensions of the Nernst and Goldman equations.
- Non-linear dynamics and complex systems theory have introduced new perspectives on how membrane potentials are established and maintained, suggesting areas where these equations could be adapted or extended.
- Interdisciplinary research combining biochemistry, physics, and computational science is uncovering new contexts and applications for these equations, from energy storage technologies to understanding the mechanisms of neurodegenerative diseases.
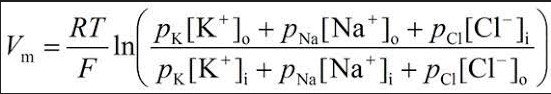
Frequently Asked Questions
What is the Nernst Equation?
The Nernst equation calculates the equilibrium potential for a single ion across a membrane, considering its concentration gradient. It’s essential for understanding how ion movement contributes to cell membrane potential and plays a pivotal role in neuronal signaling and muscle contractions.
How does the Goldman Equation differ from the Nernst Equation?
The Goldman equation expands on the Nernst equation by considering multiple ions and their relative permeabilities across a cell membrane. It provides a more accurate representation of the membrane potential, taking into account the complex interplay of various ions in cellular environments.
Why are these equations important in neuroscience?
These equations are fundamental in neuroscience for modeling how neurons generate and transmit electrical signals. They help explain the resting membrane potential and the action potentials that underlie nerve impulses, critical for brain function and sensory processing.
Can the Nernst and Goldman equations predict cell behavior?
While these equations offer valuable insights into the electrochemical aspects of cell behavior, they are part of a broader set of tools needed to predict cell behavior. They focus on membrane potential aspects, which, while crucial, must be integrated with other biological processes to fully understand cell function.
Conclusion
The Nernst and Goldman equations serve as critical tools in the exploration of cellular physiology and neuroscience, each with its unique strengths and applications. While the Nernst equation offers simplicity and specificity in analyzing single-ion effects on membrane potential, the Goldman equation provides a comprehensive framework for understanding the cumulative effects of multiple ions. Together, they enhance our grasp of cellular mechanisms and contribute to advancements in medical and scientific research.
Their enduring relevance underscores the importance of foundational principles in the rapidly evolving field of biophysics. As researchers continue to uncover the complexities of cellular function and neural communication, the Nernst and Goldman equations remain indispensable in bridging theoretical knowledge with practical applications, highlighting the beauty and intricacy of life at the molecular level.